


Next: About this document ...
Up: construct
Previous: Three Impossible Constructions
Here are a few more construction problems to try your hand at.
- Given a semicircle centered at a point
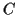 with diameter
with diameter
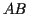 , find points
, find points 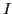 and
and  on
on 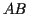 , and points
, and points 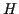 and
and 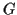 on
the semicircle such that the quadrilateral
on
the semicircle such that the quadrilateral 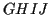 is a square.
is a square.
- Given a quadrant of a circle (two radii that make an
angle of
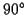 and the included arc), construct a new circle
that is inscribed in the quadrant (in other words, the new circle
is tangent to both rays and to the quarter arc of the quadrant).
and the included arc), construct a new circle
that is inscribed in the quadrant (in other words, the new circle
is tangent to both rays and to the quarter arc of the quadrant).
- Given a point
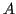 , a line
, a line 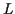 that does not
pass through
that does not
pass through 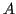 , and a point
, and a point 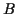 on
on 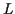 , construct a circle passing
through
, construct a circle passing
through 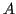 that is tangent to
that is tangent to 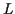 at the point
at the point 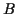 .
.
- Given two points
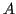 and
and 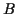 that both lie on the same
side of a line
that both lie on the same
side of a line 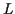 , find a point
, find a point 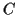 on
on 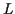 such that
such that  and
and
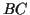 make the same angle with
make the same angle with 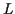 .
.
- Given two points
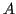 and
and 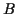 that both lie on the same
side of line
that both lie on the same
side of line 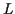 , find a point
, find a point 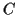 on
on 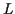 such that
such that  is
as small as possible. (Hint: This problem is related to the
construction problem 4. Also remember that the
shortest distance between two points is a line.)
is
as small as possible. (Hint: This problem is related to the
construction problem 4. Also remember that the
shortest distance between two points is a line.)
- Given two non-parallel lines
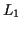 and
and 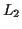 and a radius
and a radius 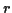 , construct a circle of radius
, construct a circle of radius 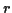 that is tangent to
both
that is tangent to
both 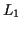 and
and 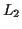 .
.



Next: About this document ...
Up: construct
Previous: Three Impossible Constructions
Zvezdelina Stankova-Frenkel
2000-11-13